The averages in maths
There are many “averages” in statistics, and the three most common are the mean, median, and mode. When you are asked to “find the average” of a set of numbers, you are commonly looking for the arithmetic mean. The mean definition is the calculated central number of a set of numbers. This means that you add up the list of numbers and then divide by the number of numbers. The median is the middle number in a list of numbers. When you are finding the median, you must list all the numbers in ascending order, and will most likely have to rewrite your list before finding the median. The “mode” is the most common number in a set of data, so if there is no number that is repeated, there is no mode in that data set. Occasionally you will also be asked for a range, which is the difference between the largest number and the smallest one.
But what does it all mean?
When you are finding the mean in maths, it is the most common average used, and it is easy to calculate. You add up all the numbers and then divide by how many numbers there are. In other words, you divide the sum of the numbers by the count of numbers.
For example, let us go through a set of numbers, and figure out how to find the average, especially considering there are a few different ways to find averages. We will find the types of mean, the mode, median, and range.
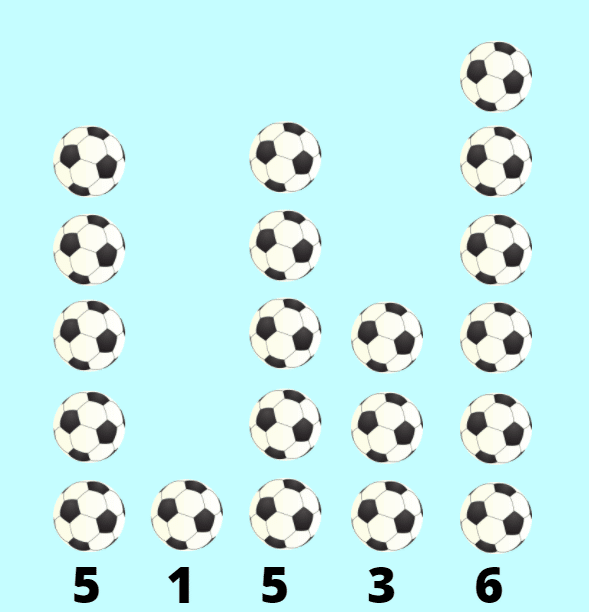
The set of data is from a group of five children juggling a soccer ball in the air using their feet. The following were the best scores: 5, 1, 5, 3, 6.
How to find the arithmetic mean:
The mean formula is the sum of the numbers divided by the amount of numbers.
Here are the steps on how to calculate the mean:
Firstly, we add the five numbers together: 5 + 1 + 5 + 3 + 6 = 20
Secondly, we divide by how many numbers there are in the data set (there were 5 children): 20 / 5 = 4
Therefore, the arithmetic mean is 4…
Even though nobody got 4, that would be the average of the class of students. This leaves a lot of students wondering “what is the meaning of mean in mathematics? How is it a value that nobody got?” And this is where the definition of mean in maths can help, as it is the calculated central value, so it may not have actually been a score that was in the data set. Another way to define mean in maths is to say, an arithmetic mean is like flattening out the numbers, so it is like all of the children in the class got 4…
How to find the mode:
Finding the mode is looking for the most common number, which in this set is quite easy, as there is only one number that is repeated, which is the number 5. Therefore, 5 is the mode. 🏅
How to find the median:
Finding the median involves looking to find the “central tendency” which is the middle number in a set.
Firstly, we must place the scores from the children in ascending order: 1, 3, 5, 5, 6
Secondly, we must look for the middle number: 1, 3, 5, 5, 6
We can see the middle number is 5.
How to find the range:
We subtract the lowest score, 1, from the highest score, 6 which equals 5.
Interestingly, the mode, range and median are 5, while the mean is 4. This then asks the question, what is the best type of average?
Well, it depends on the data set you are looking at! I would usually use the mean to find the average of anything, but I would also look at standard deviation, which is a measurement of how spread out the numbers are. As we can see from our example that nobody got 4, but the mean was 4.
The types of different “means”
Throughout school, you will likely encounter a few kinds of means when you are learning about statistics, and you will come across more in fields relating to maths, like finance, statistics and physics. Please enjoy the definition of mean in maths, for all nine of them:
- Sample mean: This is the average of a set of data. The sample mean can be used to calculate the average, central tendency, variance and standard deviation of a data set.
- Population mean: This is the average of a group-based statistic. It can be used to find out how many households own dogs in Australia.
- Mean of the sampling distribution: This is the average of the population from where items are sampled. For example, if there is a huge data set of 1,000,000 and the population distribution is normal, then the sampling distribution of the mean is likely to be normal for samples of all sizes. Therefore you could analyse data from 10,000 and assume the findings will be very similar to if you sampled the whole set of 1,000,000.
- Weighted mean: A weighted mean is similar to an ordinary arithmetic mean, but some parts of the data set contribute more than others to the total mean.
- Geometric mean: A geometric mean considers the amount of data in a set when it calculates the average for that set.
- Heronian mean: An average of two other means, the arithmetic mean and geometric mean. It arises when trying to determine the volume of a pyramidal frustum.
- Harmonic mean: This is often used when trying to find the average of things like rates. For example, the pace of a runner to do a 4km race given the duration of several athletes.
- Arithmetic-geometric mean: The arithmetic-geometric mean is used to find the values of elliptic integrals and also can be useful when on the quest to find the inverse tangent.
- Root-mean-square mean: The last of our ways to find central tendency is the root-mean-square mean. It is often used as a part of the other means, such as standard deviation. It gives a sense for the average size of data in a set of data.
What type of average will you use?
Now that you know more about the many types of averages or means, used to order and understand statistical data, you can make decisions about which of the above means are the most useful for you. You can now see that sometimes, a set of data will lend itself to be observed using a median, mean, mode, or range in the best and more accurate way. Using these different means of measurement in everyday living involves understanding which one is appropriate for each data set. Happy statistical data hunting! 🎉